Ptolemaic Astronomy

Astronomy is the oldest as well as the most prestigious of the mathematical sciences. Observing the heavens for the purposes of predicting eclipses and other phenomena occurred in the time of the Babylonians, if not earlier. Well before the beginning of the Christian era, astronomy was a demanding technical enterprise. It required long training and intense dedication of its practitioners. In this module, the computer will stand in for that training, and provide you with the theoretical toolkit possessed by a practitioner of Ptolemaic astronomy.

For much of its history, planetary astronomy, at least, has been dominated by a relatively simple set of conceptual tools. Any late-medieval or Renaissance astronomer was familiar with these tools. This module recreates the practice of astronomical theorizing pursued by such an astronomer.

What does an astronomer do, and why does he or she do it? In this period, an astronomer's duty was to predict planetary positions and eclipses. To achieve those ends, he might have to construct both observational instruments and theoretical constructs. By contrast, there were some surprising things that an astronomer did not do. He did not concern himself with the nature of the heavens and heavenly bodies themselves - at anything beyond a fairly elementary level, at least. He was regarded as unqualified to speculate extensively on the causes of celestial motions, nor, indeed, to probe far beyond the numerical figures themselves that he derived from observed planetary positions. And he was not very concerned, even within this calculational work, about the actual paths followed by the planets through the heavens. These were matters on which the mathematical techniques of astronomy could yield no certain or authoritative knowledge. They were more appropriate to the philosopher than the mathematician, since they related to real, physical entities, not abstracted, numerical ones. An astronomer was correspondingly something of a subordinate figure. His was a service industry, dedicated to providing dates and times for others of higher status (physicians, churchmen, philosophers) to put to use.

Ptolemaic astronomers thus avoided controversial speculation on the nature and mechanisms of the heavens. But their basic assumptions were nonetheless supposed to be compatible with natural philosophy -- and in particular the natural philosophy of Aristotle.

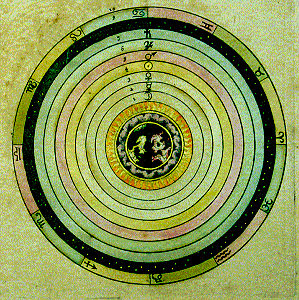As seen here in a hand-colored image from a seventeenth-century atlas, Aristotelian natural philosophy portrayed a cosmos with the Earth stationary at its center. The four elements of earth, water, air, and fire all had their own proper spheres concentric to the earth, followed by the sphere of the Moon. This marked the boundary between the "sublunary" world, in which things came into being, changed, and died, and the "superlunary" realm, in which things were eternal. Beyond lay the planets, or "wandering stars," which moved around the earth in perfect spheres. The sphere of the fixed stars contained all of these, with nothing beyond it except God. Christians changed the gloss slightly to assert that the cosmos as a whole was not eternal - it had been created by God, they insisted, and would eventually suffer annihilation at his hand - but they kept the natural-philosophical principles largely intact. On this basis, Christianized Aristotelianism provided coherent and largely convincing knowledge of natural processes for some 450 years, from the reintroduction of Greek philosophy in around 1200 to its eclipse in the "Scientific Revolution" around 1600-1650.

But astronomers were not philosophers. They accepted the basic structure of the Aristotelian cosmos, but did not see their task as one of explaining its nature. Their role was to predict significant celestial events (like eclipses and conjunctions ), provide astrological forecasts, and identify propitious days on which to administer medicines. For such purposes Aristotelian cosmology proved not so much inadequate as inappropriate. Astronomers instead developed their own, rich, mathematical tradition. But the result was a multiplicity of different theories, all unique, but all properly called "Ptolemaic" because they embodied the theoretical devices of the Almagest.

What was it like to pursue this kind of enterprise - an enterprise very different from the modern science of astronomy, and yet of which that modern science is the descendant? In this module you are given the chance to find out. You yourself become a Ptolemaic astronomer. The computer provides you with the theoretical armoury an astronomer possessed as a result of his training at university or through dedicated reading. You are faced with an observed path of a planet. Your task is effectively that faced by any astronomer of the Middle Ages or Renaissance seeking to placate his prince or advance in his university. Can you achieve success?

Ptolemaic Astronomy: Some Conclusions

You have probably left the simulation with your theoretical model matching the observed motions well in some places, but with distressing divergences in others. In that, your experience has corresponded fairly well to those of Ptolemaic astronomers in medieval Islam and Renaissance Europe. Exercising the kinds of judgment they exercised should also have served to fix in your mind some characteristics of astronomy as it was then defined that may seem puzzling to a modern user. And it may well have raised questions that would not have occurred to a real Ptolemaic astronomer at all. These questions reach to the very definition of astronomy - and they profoundly affect how we think of its history.

Theory Construction: Is Empiricism Enough

One of the most difficult practical questions for an astronomer doing this kind of work was this: when do I stop? By combining equant, deferent, and epicycle, you will be able to match the motions of a planet fairly closely. But how closely is sufficient? And how do you know that the degree of approximation you choose is the right one? Bear in mind that much may hang on your decision, from the date of Easter to the moment when you take your medicine. In fact, the decision to stop doing astronomy marked the moment when a new theory came into being.

But this was not the end of the problem. Not only was the appropriate degree of exactitude obscure; there were also an indefinite number of ways of achieving it. With Ptolemaic assumptions and tools, an astronomer could "save" the appearances of a planet in an infinite number of ways. Who was to decide which of these ways should be preferred, and be accounted an astronomical theory? On what grounds?

It is particularly evident, then, that given a practice of this kind, the knowledge that Ptolemaic astronomers prized as their achievement was of a peculiar sort by the lights of modern science. It could be very precise, and accurate to the nth degree, and there was widespread agreement about the legitimacy of Ptolemaic assumptions in general - yet there was no guarantee as to the physical truth of any theory in particular. This reflects very important characteristcs about both the natural world of the Renaissance and the social world of the astronomers.

In the sixteenth century awareness of such conundrums gave rise to fierce controversy. Nicolaus Copernicus wrote De Revolutionibus (On the Revolutions) out of conviction not only that the earth was in motion, but that certain knowledge of astronomical motions was possible.

What is an Orbit

Ptolemaic astronomy could produce a planetary trace matching observations in any number of ways. But the theories might not be equivalent after all. The actual paths followed through space by the planets carried on all those epicycles and deferents differed widely. Some of these planets had orbits so bizarre that they could not really be accounted orbits at all. Could this not provide grounds for choosing between rival proposals?

In practice, it often could not. The reason for this lies in what Ptolemaic astronomers knew about both the physical and the social worlds.

It seems obvious today that mathematics has a principal part to play in understanding natural phenomena. But in Renaissance Europe this was widely doubted. Aristotelian philosophers in particular tended to regard the making of claims about nature on the basis of mathematical arguments with suspicion. It seemed to them to embody a fundamental category mistake. The point of natural philosophy was to consider nature in its substantial whole, and to provide causal accounts of why phenomena occurred as they did; mathematics treated quantity - at best but one aspect of such phenomena - and had nothing to say on the crucial matter of causation. The mathematical sciences were therefore "mixed," in that they applied mathematical techniques to inappropriate, non-mathematical objects. They might produce tangible effects, but not real philosophical knowledge.

Most Ptolemaic astronomers would have agreed. Theirs was a mathematical enterprise. That is, it did not advance philosophical claims about the nature of the heavens, the heavenly bodies, and their motions. It was not that they did not see the value of seeking the truth about such matters; solely that mathematics was not the enterprise for those doing so. The right enterprise for such seekers was natural philosophy. Natural philosophers were thus more prestigious than astronomers (and all other mathematicians). They received greater salaries and more renown.

Finding out about Ptolemaic practice thus not only reveals important information about astronomy - showing what a different enterprise it was in the Renaissance from the science that now goes by the same name. It also tells us something important about the natural philosopher's role, too. And it shows how those two distinct disciplines resulted in the nonexistence of something that to us seems a self-evident presence in the world. In fact, it is not just that the more crazy planetary paths generated by Ptolemaic mechanisms cannot be accounted orbits. None of the paths are orbits. Even when Copernicus published his De Revolutionibus, working astronomers could comfortably transform his models into a system with a stationary Earth because for someone pursuing this practice the reality of these paths required no commitment. Only when Johannes Kepler overthrew the entire practical enterprise of Ptolemaic astronomy did the concept of an orbit begin to have some meaning. Yet even Kepler owed something to Ptolemy. He rejected existing astronomical theories - and the entire enterprise of which they were the product - partly by reviving a very ancient idea that the universe must exhibit harmony, and by insisting that it was his role to understand this harmony. And Kepler was convinced that Ptolemy had known this long before him. He tried hard to recover and complete a long-neglected text by the ancient astronomer on the subject of harmony itself - a text which, he believed, would reveal the commitment of Ptolemy to identifiably similar views. The ravages both of time and of the religious wars of Kepler's own age foiled this plan to revive Ptolemy for yet another age.